The Speed of Gravity - Repeal of the Speed Limit

Abstract. The mathematical equations of general relativity are unique, but their physical interpretation is not. Confusion reigns over the difference between the field and geometric interpretations of GR, the meaning of gravitational force in a GR context, the distinction between gravitational waves and force variations, and the applicability of aberration to gravity. The geometric interpretation of GR, argued by Carlip, blurs these concepts. We show that aberration has been suppressed in the GR equations of motion through setting gravity’s propagation speed to infinity; and that the absence of aberration cannot be explained through some mathematical “cancellation” because that would cancel tidal forces too. The mere existence of Lorentzian relativity as an experimentally viable model for the relativity of motion nullifies the “proof” that nothing can propagate faster than light in forward time. Experiments indicate that gravity and electrodynamic forces both propagate far in excess of lightspeed. Short abstract. General relativity has a geometric and a field interpretation. If angular momentum conservation is invoked in the geometric interpretation to explain experiments, the causality principle is violated. Meanwhile, the field interpretation avoids this problem by allowing faster-than-light propagation in forward time. Lightspeed is not a universal speed limit.

 

1. Experiments measuring the “speed of gravity”

We recently reviewed six experiments bearing on the question of the propagation speed of gravity. [1] Briefly, these are:

(1) a modern updating of the classical Laplace experiment based on the absence of any change in the angular momentum of the Earth’s orbit (a necessary accompaniment of any propagation delay for gravity even in a static field);

(2) an extension of this angular momentum argument to binary pulsars, showing that the position, velocity, and acceleration of each mass is anticipated in much less than the light-time between the masses;

(3) a non-null three-body experiment involving solar eclipses in the Sun-Earth-Moon system, showing that optical and “gravitational” eclipses do not coincide;

(4) planetary radar ranging data showing that the direction of Earth’s gravitational acceleration toward the Sun does not coincide with the direction of arriving solar photons;

(5) neutron interferometer experiments, showing a dependence of acceleration on mass, and therefore a violation of the weak equivalence principle (the geometric interpretation of gravitation); [2]

(6) the Walker-Dual experiment, showing in theory that changes in both gravitational and electrostatic fields propagate faster than the speed of light, c, a result reportedly given preliminary confirmation in a laboratory experiment.

To these, we can now add:

(7) An earlier laboratory experiment ([3], with summary description in [4]) showed that charges respond to each other’s instantaneous positions, and not to the “left-behind potential hill”, when they are accelerated. This demonstrates that electrodynamic forces must likewise propagate at faster than lightspeed more convincingly than earlier experiments showing angular momentum conservation.

(8) A new laboratory experiment at the NEC Research Institute in Princeton claims to have achieved propagation speeds of 310 c. [5] This supplements earlier quantum tunneling experiments. [6] It is still debated whether these experiment types using electromagnetic radiation can truly send information faster than light. [7] Whatever the resolution of that matter, the leading edge of the transmission is an electromagnetic wave, and therefore always travels at lightspeed. However, such experiments have served to raise public consciousness about the faster-than-light-propagation concept.

Of all these experiments, #(2) -- the binary pulsars -- places the strongest lower limit to the speed of gravity: 2x1010 c.

2. Principles and definitions

Mathematics and physics take fundamentally different approaches to describing nature. The former is more concerned with what might be possible, and the latter with what is definitely real. Math is constrained by the need for internal consistency, but is generally oblivious to external constraints. Physics has its laws too, and these can change as knowledge improves. But physics is rigorously constrained by its principles, which have no counterparts in mathematics. [8] Examples are the causality principle (“every effect must have a proximate, antecedent cause”) and the prohibition against “creation ex nihilo”. Violations of such principles are ruled out by logic as requiring magic, a miracle, or the supernatural. Although mathematically allowed, they are said to be “physically impossible”.

A specific example where math and physics take different paths, relevant to this article, is the matter of gradients. Consider the gradient of gravitational potential, where potential is a scalar quantity. In math, the gradient of a scalar field is a unique, unambiguous thing; for example:

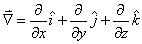
Note that the gradient of a scalar field is a vector, not another scalar. But if the field source begins to move, does the field gradient point toward the instantaneous or retarded position of the source? That depends on whether the field updates or regenerates instantly or with delay. So when we say that the gravitational acceleration of a test body follows the field gradient, we must ask which gradient it will follow -- instantaneous or retarded. Physics has an issue that math does not. Retarded potentials in math allow for delays only in the mass distribution and in changes of distance between masses in a scalar field. Retarded potentials in physics must allow also for delays in the vector direction of the field – normally the dominant effect of retardation.

A corollary of the causality principle is the prohibition of true “action at a distance”, because every effect must have a proximate cause. That means that something (call it an “agent”), whether particle or wave or wavicle or other, must pass (or fail to pass) between a source of gravity and an accelerated target to produce the acceleration. Moreover, this agent is the carrier of the momentum transferred between source and target. In math, momentum transfers to the target could take place in any effective direction. We need merely write an equation to describe which one. But in physics, the only available source for the momentum transfers is the actual momentum of the agents that communicate between source mass and target body. And the speed and direction of travel of those agents (necessarily radial from a monopole source mass) comprise the velocity vector portion of the momentum transferred to the target. In physics, no freedom to choose another direction exists unless a secondary cause with a force of its own is introduced.

The matter of definitions of terms is also important. In math, one can associate a mathematical symbol with a physical concept, then manipulate symbols and derive new information about the concept. But in physics, definitions must connect concepts to observations or experiments. For gravitation, this difference of approach has led to two different interpretations of GR: the “field” interpretation with forces and motions through 3-space; and the 4-dimensional “geometric” interpretation in which “gravity is just geometry” without need of forces as such. Einstein and early relativists dealt with both interpretations as if they were interchangeable; but in the context of this paper, we will see that they are not.

In modern times, professional relativists have come predominantly from the ranks of mathematicians, resulting in the almost exclusive ascendancy of the geometric interpretation. This viewpoint has been carried to its extreme form in modern GR textbooks such as that of Wald, who denies the viability of the field interpretation altogether: “The basic framework of the theory of general relativity arises from considering ... that we cannot in principle -- even by complicated procedures -- construct inertial observers in the sense of special relativity and measure the gravitational force. This is accomplished by the following bold hypothesis: The spacetime metric is not flat, as was assumed in special relativity. The world lines of freely falling bodies in a gravitational field are simply the geodesics of the (curved) spacetime metric. In this way, the ‘background observers’ (geodesics of the spacetime metric) automatically coincide with what was previously viewed as motion in a gravitational force field. As a result we have no meaningful way of describing gravity as a force field; rather, we are forced to view gravity as an aspect of spacetime structure. Although absolute gravitational force has no meaning, the relative gravitational force (i.e., tidal force) between two nearby points still has meaning and can be measured by observing the relative acceleration of two freely falling bodies. ...” [9]

At the other extreme, Feynman preserves the force and motion concepts with their classical meanings, and comments: "It is one of the peculiar aspects of the theory of gravitation, that it has both a field interpretation and a geometrical interpretation. ... the fact is that a spin-two field has this geometrical interpretation: this is not something readily explainable – it is just marvelous. The geometrical interpretation is not really necessary or essential to physics.” [10]

In this paper, we will argue that the geometric interpretation violates the causality principle, and is therefore falsified in physics. (Of course, it remains mathematically valid.) To that, we can add the results of the neutron interferometer experiments [2] that purport to falsify the geometric interpretation because a dependence of acceleration on the body’s own mass is demonstrated. We will therefore follow the field interpretation herein, defining our terms in the traditional physics way.

The standard GR equations of motion [11] are expressions for gravitational 3-space acceleration – the second derivative of the position vector with respect to time. (“Time” here means “coordinate time” in a relativistic context.) Multiplying acceleration by target body mass (a constant) yields an expression for what we may call "gravitational force" by definition. If we integrate this force expression with respect to radius vector r (instantaneous distance from source mass to target body), we arrive at an expression for what celestial mechanicians call "gravitational potential". For the gravitational field, this makes gravitational force the instantaneous gradient of gravitational potential. These are all standard concepts in relativistic celestial mechanics.

GR involves gravitational source masses and their fields. If a source mass and its field move in lockstep to infinity, so that whatever happens to the source happens to the whole field instantly in coordinate time, that is defined to be “instantaneous” propagation of the gravitational field. But if a change in the motion of a source mass results in a delayed corresponding change in its field, then the propagation of that field is said to be “retarded”. If ambiguity arises, the appropriate coordinate time is that of a clock at rest with respect to the center of mass of the system under consideration, but infinitely far removed from the gravitational influence of those masses.

3. Gravitational waves and force variations confused

Because no experimental data suggests that gravity might propagate as slowly as lightspeed c, most of the debate revolves around confusion over the meaning of the expression “the speed of gravity”. It is undisputed that gravitational waves, assuming these entities exist, must propagate at lightspeed c. However, gravitational waves are still hypothetical, yet are often confused with changes in gravitational fields (“force variations”). The existence of the latter is firmly established as they are detected daily with gravimeters. If a massive body of stellar dimensions can generate a significant gravitational wave (e.g., by explosion), that wave would still be ultra-weak, and unable to contribute to the acceleration of any other astrophysical body at a presently detectable level. Indeed, our most sensitive detectors have not yet succeeded in identifying gravitational waves from any astrophysical source, despite indirect evidence for their existence from binary pulsars. Would-be gravitational wave detectors seek motions that are only a fraction of an atomic diameter.

By contrast, gravitational fields and field variations produce large accelerations of major astrophysical bodies. If our Sun were a double star, the easily detected force variations emanating from the pair would not win anyone a Nobel Prize for the first detection of gravitational waves. Changes in gravitational fields, whether themselves of wave-like character or not, are a distinctly different phenomenon from the “gravitational waves” of GR. For example, a neutron star binary pair might have an orbital period as short as a millisecond, producing force variation cycles every half millisecond. Photons and gravitational waves travel 150 km in a half millisecond, so different delays in their arrival time at various detection stations will be seen. But several of the experiments (1)-(6), especially (2), show that force variations have no propagation delay at all comparable to that of light. The gravitational maxima of the neutron binary would be quite out of phase with the optical eclipses and gravitational wave cycles because of differing propagation speeds for gravity and light, just as was shown in experiment (3).

Moreover, gravitational fields and changes in those fields (due to acceleration of a source mass) all emanate from the source of gravity. By contrast, gravitational waves emanate from a target of gravity as it is forced to accelerate for any reason, as in the case of a supernova. (The gravitational field of a supernova changes only relatively slowly for a spherically symmetric explosion.) Gravitational radiation (= waves) arises from a body’s inertial mass, not its gravitational mass. This is because it is determined by the permeability and permittivity of the “spacetime medium”, rather than by the curvature of that medium. Gravitational waves are therefore an effect of acceleration, not a cause; and would presumably be generated even by two hypothetical non-gravitating orbiting masses held in place by a connecting tether. (Because Einstein’s equivalence principle equates these two types of mass for all bodies, such a hypothetical example might be impossible in the physical world.)

Correspondingly, propagation delays for gravitational force (or whatever produces gravitational acceleration in 3-space) would cause orbits to gain angular momentum and expand at a rate independent of the mass of the orbiting body; whereas radiation of gravitational waves causes orbits to lose angular momentum and shrink at a rate that does dependent on the mass of the orbiting body.

In summary, we can use the analogy of a buoy (Figure 1). If the anchor holding the buoy in place is moved, the chain connecting them causes the buoy to move. This is analogous to gravitational force variations between a source mass (anchor/Sun) and a target body (buoy/Earth). As the buoy moves, it sets off water waves. These are analogous to gravitational waves, which disturb “spacetime” (or “the light-carrying medium”, whatever its nature). The anchor or source mass is a cause of changes in target motion; the water waves are an effect of those changes.

S. Carlip has now also commented on our previous paper. [15] Carlip argues for the consistency of some of these experiments with the geometric interpretation of general relativity, assuming that gravity propagates at lightspeed. However, neither experiment (5) or (6) on the above list (if independently verified) is consistent with the geometric or lightspeed interpretations of GR, although they are consistent with the field interpretation of the same equations in flat space-time. [16,17] In brief, Carlip (following recent practice) repeatedly blurs the distinction between changes in gravitational fields and gravitational waves, thereby arriving at conclusions applicable only to the latter, but claiming they also apply to the former.

4. Comparing gravitational and electromagnetic (light) fields

As explained with diagrams and equations in our previous paper [1], aberration is the change in the effective direction of approach to a moving target necessitated by the propagation delay of any projectile (whether particle, wave, wavicle, or other). We apply that here to two different types of field-generating forces: gravitation and the radiation pressure from sunligh

If gravity and light originate simultaneously at the same source (e.g., the Sun) and propagate to the same target (e.g., the Earth) at the same speed (e.g., c), then aberration will be the same for both. (That is because tan (aberration) = vorbit /Vpropagation where vorbit is the transverse orbital speed of the target body and Vpropagation is the propagation speed of field regeneration. Approximately, aberration vorbit /Vpropagation.) Therefore, gravity and light should produce 3-space accelerations in the same direction (or exact opposite direction, if push and pull are reversed), differing only in magnitude by a scaling constant. Specifically, the momentum transferred to or removed from the target to produce the acceleration is some constant (usually a mass) times Vpropagation, a vector, in both cases.

The only physical way to break this equality would require postulating some source of the momentum transfer other than, or in addition to, the propagation speed of the field. Such a postulate would seem to constitute new physics, and would appear to be in trouble with the causality principle because radial forces from the source mass are already spoken for. In the absence of any serious such proposal for a new force, and given that gravity and radiation pressure do not produce anti-parallel forces in experiment (4), one of our premises must be violated. The only premise open to question is the equality of propagation speeds of the two types of force. If gravity propagates very much faster than light, all experimental evidence is immediately satisfied.

We see that all relevant characteristics except possibly field propagation speed are common for the two types of force. Therefore their physical propagation behavior ought to be the same if field propagation speed is the same, or different if field propagation speed is different, because there is nothing else relevant that might distinguish the two types of force. Experiments show without ambiguity that the resulting accelerations are applied in different directions, which implies different field propagation speeds.

To clarify, note than an accelerating target is necessarily changing its momentum vector. Momentum is the product of mass times velocity (or energy over c2 times velocity, if the projectile is massless) for whatever “projectile” carries the force from source to target. The "velocity" in the momentum is the velocity vector of the carrier, whose direction of travel is along the radial from source to target. Because the momentum is necessarily directed along the radial direction, no part of the exchanged momentum could be applied in some other direction. Specifically, if the projectiles are photons and the force is radiation pressure, the target is pushed in the direction of the arriving photons. Whatever is the mechanism of gravity, how could the direction of its pull be other than along the radial to the source mass? The physics and the meanings of words such as "momentum = mass times velocity" dictate only a single possibility for the vector direction of momentum transfers: the radial direction between source mass and target body. And if that is true, the only physical way left to explain the different effective direction of the force is a different propagation speed for a retarded field.

The same remarks apply to electrodynamic forces between charges. If the field propagation speed were lightspeed, we would be forced to interpret the momentum transfers as applied in a different direction from the direction of travel of the momentum carriers because of aberration. That is why, when the details are examined closely, we hear statements such as “virtual ‘photons’ (the hypothetical carriers of electrodynamic forces, not to be confused with real photons) travel at infinite speeds”. So this "lightspeed propagation" assumption is unphysical and unnecessary. One can have the same equations (to the accuracy of observations) and interpret them in a physically consistent way if the momentum carriers travel much faster than light.

Now that we know that Lorentzian relativity is experimentally viable [18] and allows faster-than-light (ftl) propagation in forward time [19], ftl propagation is no longer forbidden in physics, and ftl force carriers are the most reasonable interpretation of the equations. I expect that no one would ever have thought otherwise if they had not mistakenly believed that ftl propagation was forbidden in physics.

If the field around a charge propagated with lightspeed delays, then Maxwell's equations would be wrong because they neglect transverse aberration, the main manifestation of field propagation delay. The fact that the equations are correct to first order in v/c tells us that the field propagation speed must be very fast compared to lightspeed, because the neglect of transverse aberration is the logical equivalent of adopting infinite field propagation speed. If Maxwell's equations did work for fields propagating at lightspeed, then they would work for radiation pressure from light fields too, which they do not. If the field has a measurable delay in reshaping itself to register the motion or acceleration of its source, then one would need to add propagation delay into Maxwell's equations (for example, by taking partials with respect to retarded coordinates instead of instantaneous coordinates). The absence of such propagation delay in the equations means that instantaneous propagation of fields is already built in.

5. How the “speed of gravity” appears in the GR equations of motion

The speed of light, c, appears in the Schwarzschild solution to the Einstein field equations, and in the GR equations of motion. It plays the dual role of permeability or permittivity of space-time (a measure of its resistance to curvature) and as the wave speed in the equation for gravitational waves. It appears in all GR terms in n-body equations of motion beyond the basic Newtonian acceleration terms. See, for example, Misner et al. [11], where c has been normalized to unity; or the source of those equations, Einstein et al. [20] or Robertson & Noonan [21], where c is retained explicitly.

These equations are lengthy because they are for n bodies and include higher-order terms in an infinite Taylor series. For our purposes here, only terms that are first-order in potential or first- or second-order in velocity are relevant. The sum of all higher-order terms to infinity is negligible for all astrophysical cases under consideration. This allows us to ignore most of the terms in the equations of motion as insignificant for this discussion, allowing a great economy of form and ease of understanding.

Newton’s general formula for the gravitational acceleration of a planet of negligible mass in the field of the Sun is

(1)

where 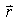is the vector from Sun 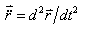to planet and r is its magnitude; G is the universal gravitational constant; M is the mass of the Sun; is the 3-space acceleration vector of the planet relative to the Sun; and t is (“coordinate”) time. [22] Without directional information (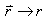), this would reduce to the more familiar inverse square form. But we need directionality in this discussion.

The GR equations of motion are taken from Misner et al. [11], with notation adjusted to agree with the above conventions. We now need additional definitions. is the 3-space velocity vector of the planet relative to the Sun; (as before) c is the speed of light, and 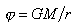is the scalar gravitational potential of the planet in the Sun’s field. Then the GR equations of motion for a planet in the Sun’s field are

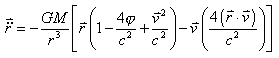(2)

A careful examination of Einstein et al. [20] or Robertson & Noonan [21] would yield the same expression. These terms are sufficient to produce solar light-bending, redshift, radar time-delay, and perihelion advance, the classical effects that differentiate GR from Newtonian gravity. Note that this equation reduces to the Newtonian law in the low velocity , weak field limit. In the geometric interpretation of GR, the new terms that appear here represent “space-time curvature”. They have nothing to do with the speed of gravity.

In both the Newtonian and the GR equation of motion, all quantities take on their instantaneous values for any given time t. No one disputes that Newtonian gravity has infinite propagation speed built in. [11] In GR, one of several ways to get equations of motion is to form a Hamiltonian (an expression for the total energy, potential plus kinetic, for a system of bodies), and take partial derivatives with respect to some chosen coordinates and momenta. In this crucial step for GR, the partials are always taken with respect to instantaneous, rather than retarded, coordinates and momenta, thereby neglecting aberration and implicitly adopting instantaneous gravity. Retarded values are not used because then the equations of motion would no longer conserve angular momentum; i.e., they would be wrong. But the logical consequences of this choice have seldom been elaborated.

To explicitly see the effect that a finite speed of gravity would have, we can parameterize it. Let the speed of gravity be V. Then the propagation time for gravity would be r/V, a small quantity. Because this propagation delay time is short, we can save unneeded work in our derivation by taking the motion of the planet during the gravity propagation time to be linear, and by ignoring small changes in already small quantities such as or 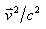. Therefore, the only quantity that changes significantly during the gravity propagation time is 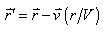, where prime ( ' ) denotes a gravity-propagation-time retarded value.

We can spare ourselves additional small, unneeded terms by considering only the transverse motion of the planet, ignoring radial effects (which are smaller than the terms we keep by a factor of the eccentricity of the orbit). This allows us to use the approximation 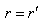; i.e., the Sun-planet distance has little or no change, although its direction still changes rapidly. That will restrict our focus to transverse velocity, which is the quantity that gives rise to transverse aberration. With this simplification that changes nothing material to this discussion, Newton’s retarded gravitation law

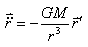
becomes

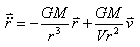(3)

This is Newton’s law with the speed of gravity explicitly shown. Obviously, this new term vanishes as the speed of gravity 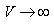, which shows that we recover the normal Newtonian law under that condition, as expected. Note, however, that we already have a potential problem with the geometric, lightspeed interpretation of GR. In the weak-field, low-velocity limit, if we claim that gravity propagates at the speed of light, the new term in equation (3) will not vanish.

To introduce the speed of gravity into the GR equations, we make the same substitution of retarded for instantaneous values. Not surprisingly, only the Newtonian part of the GR equations of motion is large enough and changes fast enough that retardation is significant. We therefore end up with the same new term.

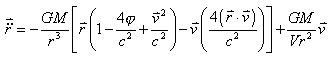(4)

These are the GR equations of motion with the speed of gravity 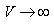retained explicitly. This new term still goes to zero as the speed of gravity , in which case we recover normal GR. To recover Newtonian gravity from this equation, we would also have to set the “space-time curvature” or “permeability of space” that characterizes GR to zero by letting 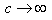. The consequences of setting c to infinity or V to infinity should not be confused. It should also be noted that the speed of gravity term is proportional to v/V (first order in velocity), whereas the most similar “curvature” term is proportional to v2/c2 (second order in velocity). In the most favorable comparison case, V = c, the latter term is still much smaller than the former. For typical solar system cases, the difference is four orders of magnitude. In short, second and higher-order terms in v, even summed to infinity, are too small to affect this discussion of the speed of gravity.

The assumption that gravity propagates at lightspeed would change the GR equation of motion to eqn. (4) with V = c, which is observationally unacceptable. It is therefore without doubt that gravity has no detectable propagation delay or aberration. Only the implications of that fact are in dispute. The statement that “the speed of gravity equals the speed of light” is manifestly false, and is heard often only because of the confusion with the propagation speed of gravitational waves.

6. Retarded potentials and gradients

In example 1, the gradient cannot point toward the new position of M until the adjustment wave has time to propagate from M to P. However, let’s now consider the case where M remains fixed on the rubber sheet, and P has the transverse motion. Then the direction of the gradient would once again point toward the instantaneous position of M. Call this "example 2". In this case, the gradient direction is independent of the transverse motion of P; i.e., it has no aberration. This is what the geometric interpretation of GR proposes.

In this example 2, nothing propagates from M to P. So aberration is not applicable, and apparent agreement with experiment is secured. Note that this agreement does not result from a propagation speed of c, but from no propagation at all. This is a case of a mathematically elegant solution that is physically impossible as an explanation for gravity. A gradient, a slope, or a curvature cannot induce a body at rest in a field to begin moving unless a force acts on that body. This is because, without a force to initiate motion, the body has no reason to choose any one direction over any other direction. Moreover, momentum would not be conserved if the field had no moving parts, yet a body at rest in it began moving in some direction without a force acting. (Recall that, by "force", we mean a "transfer of momentum".)

In the rubber sheet analogy, if the small target body is at rest on the side of a dent caused by a source mass, it will remain at rest forever until some force acts. The rubber sheet analogy works in our imaginations only because we instinctively imagine gravity under the rubber sheet, with its pull providing a meaning to the concept of “downhill”. But without pre-existing gravity, the small body has no cause to accelerate, either on the rubber sheet or in the geometrical interpretation of GR. The causality principle is violated.

So how do real forces propagate? The field interpretation still provides the best answer. The change from field equations to equations of motion is analogous to going from a potential to a force by taking a gradient. The process of "taking a gradient" (or taking partial derivatives in general) is unique and unambiguous only after one has decided the issue under discussion here -- whether the distant fields are in accord with the instantaneous or retarded directions of their sources. This choice has an immediate, first-order effect on the direction of the resulting force -- by the amount of the angle of aberration.

Over 100 years of history back the choice of instantaneous fields as the observationally correct one. However, it is still wrongly claimed that instantaneous fields are consistent with force propagation at lightspeed. Lightspeed propagation requires choosing retarded fields and field directions. Note the emphasis on directions. Using retarded distances or matter distributions is unimportant for our purposes here because their effects are negligible in comparison to the retarded source mass direction effect.

As an example, let’s compare two different fields. The Sun emits light, just as it emits gravity. Light forms an inverse square field around the Sun, just as gravity does. For the gravitational field, gravitational force is the gradient of gravitational potential. Analogously for the light field, light intensity is the gradient of light amplitude. For a non-moving observer, we could do a one-to-one mapping of potential and amplitude, or of force and intensity. In either case, the difference everywhere is a constant scale factor. If light or gravity propagated instantaneously, then an observer on Earth orbiting the Sun would detect that instantaneous field. However, the combination of a finite field propagation speed and an orbiting observer would make the field appear retarded as seen by the observer.

Specifically, in the case of light, which unquestionably has a finite propagation speed, light intensity drops off with the inverse square of distance along a radial from the retarded position of the Sun. Note that this is a different direction in space than the field direction seen by a non-moving observer. Nonetheless, it is a real directional difference, not an optical illusion. The Sun not only looks to be in a different place among the stars, but the momentum transferred to Earth by the pressure of sunlight, the radiation pressure force, acts along the radial from the retarded Sun, not the radial from the true Sun. This is the well-known “Poynting-Robertson effect” – too weak to move planets, but very effective on dust particles and balloon satellites. Correspondingly, the gradient of the amplitude for this field as observed from orbiting Earth points toward the retarded Sun, not the instantaneous Sun.

By analogy, if gravity propagated at the same speed as light, the gradient of the potential of the gravitational field, as seen by an orbiting observer, would point toward the retarded position of the source, not its instantaneous position. Whether or not something later cancels this retardation effect, it cannot be omitted as the opening step in describing the gravitational field. When taking the field gradient to get the direction of gravitational force, or when taking partial derivatives to derive equations of motion, if the field has a finite propagation speed, such gradients and partials must respect the retarded directionality of the field for a moving observer. The fact that the gradients and partials actually used in GR ignore transverse aberration, the chief effect of propagation delay, is the logical equivalent of adopting instantaneous gravitational field propagation in GR.

We should not be fooled by consideration of retardation in the distance or mass or charge distribution, a common practice in textbooks on electromagnetism and gravitation. For example, let a scalar potential be given by GM/r, which has a magnitude but no direction. Retardations in the value of r are of almost no consequence because (1) they are often small and slowly changing (e.g., for near-circular orbits); and (2) they give rise to only periodic effects in orbits. Similar remarks apply to retardation of changes in mass or charge distribution.

By contrast, the gradient of the potential has magnitude and direction. For example,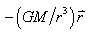 is the gradient of the potential just given. Again, retardation in r is relatively unimportant. But retardation in 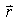is all-important in this context because (1) it is of order v (the velocity) to the first power, not diminished by eccentricity or any other parameter; and (2) it gives rise to secular (i.e., progressively increasing) effects that can eventually exceed any threshold for observational detection.

Retardation in 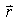gives rise to the Poynting-Robertson force when we are dealing with radiation pressure. Retardation in would also be the largest consequence of gravity or electric force propagating at a speed as slow as c. But no such retardation terms appear in either Maxwell's or Einstein’s equations. The absence of such terms is the logical equivalent of setting the field propagation speed to infinity, which drives the first-order-in-v term to zero.

At this point, we have shown that:

· Transverse aberration must always be considered when transverse motion of the target body occurs.
· Specifically, transverse aberration is present for light, a field propagating at the speed of light.
· Aberration must also apply to transverse motions through electrodynamic or gravitational fields.
· The absence of aberration in the equations of motion is the logical equivalent of taking the field propagation speed to be infinity, because that drives aberration to zero.
· Strongly faster-than-light (ftl) propagation speeds violate no laws of physics because Lorentzian relativity (LR) is experimentally viable and allows ftl propagation speeds; and the field interpretation of GR is as compatible with LR as it is with SR.
· So ftl propagation in forward time is definitely possible; and gravitational and electrodynamic forces are examples of such ftl propagation.

7. Carlip’s paper

In the geometric interpretation of GR, gravity is not a “force” and cannot propagate because target body motion simply follows a curved geodesic path through “space-time” without any force acting. Experiment (5) disputes the possibility that this forceless interpretation of gravity could be correct. So does the dependence of the full GR equations of motion for a target body on that body’s own mass. Both are failures of the weak equivalence principle – the notion that “gravity is just geometry”.

In his paper [15], Carlip recognizes the concept of gravitational force, as in the field interpretation of GR. But he claims that the experiments (1)-(4) are not direct propagation speed measurements for gravity. They are, however, measurements of aberration or v/V, where the only unknown is the speed of gravity, V. This makes Carlip’s point semantic because the “indirectness” of these measures is not significant.

Carlip claims that we have made an implicit assumption that gravitational acceleration is central and has no velocity-dependent terms. If aberration were non-zero, the acceleration would be non-central. However, observations all confirm that it is in fact central to order v/c; i.e., directed along radials from the source mass. GR implies that gravitational acceleration is target-body-velocity dependent at order v2/c2, as shown in equations (2) and (4); but velocity-independent to order v/c. It is also source-mass-velocity dependent in some of the small terms we omitted. However, all these velocity-dependent terms are typically orders of magnitude too small to cancel the aberration term if V = c. So Carlip’s point is again irrelevant to this discussion because no velocity-dependent terms exist at first order in v.

Our problems with Carlip’s equations begin with his (1.6). This is missing transverse aberration, the largest manifestation of propagation delay. That is obviously a critical error for such an early step. If one never considers genuine retardation of propagation between source mass and target body, then infinite propagation speed for gravity is being assumed, whether one says so or not.

Carlip considers his key starting point to be his equation (1.8). Its first term, proportional to ni, represents the transverse aberration. The second term, proportional to vi, cancels this transverse aberration to first order. Similarly, in the GR part of the paper, the corresponding equation where this cancellation occurs is (2.2). These two formulas are the only places where transverse aberration enters Carlip’s discussion. However, the alert reader will see that Carlip is beginning with a force vector that points closely toward the true, instantaneous position of the source (to first-order in v), when he should be beginning with a retarded position. In other words, he assumes what he proposes to demonstrate. Obviously, a vector toward the instantaneous source position can be decomposed into an arbitrary pair of component vectors. So it is unremarkable that Carlip decomposes the instantaneous position vector into a retarded position vector plus an update vector for motion during the propagation delay. This merely creates the illusion that he has begun with a retarded position vector, when in fact he has begun with a (nearly) instantaneous position vector formed of two components.

By omitting transverse aberration, Carlip (like many before him) has effectively adopted a field propagation speed 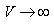. No physical or observational basis exists for postulating V = c.

8. Angular momentum and tidal friction

If gravitational aberration were non-zero, the angular momentum of an orbit would progressively increase with time, an effect that is not observed. Real orbits conserve orbital angular momentum to the accuracy of all observations, except when tidal or non-gravitational forces operate. Carlip claims that the conservation of angular momentum needed by GR justifies the cancellation of transverse aberration. He claims this cancellation is not magical, but arises naturally in the mathematics when one imposes angular momentum conservation.

It is true that imposing angular momentum conservation cancels aberration, but that begs the question. What physical justification exists for simply imposing orbital angular momentum conservation into equations when orbital angular momentum is not conserved by nature for other types of force? McCarthy [23] has pointed out that, to the Moon, the offset of the direction of Earth’s gravitational force due to tidal friction is indistinguishable from the offset of the direction of Earth’s gravitational force due to aberration. So if gravitational aberration exists after all because gravity propagates at lightspeed, but is cancelled by a velocity-dependent force provided by nature to conserve angular momentum (as Carlip claims), then nature must also cancel tidal friction because it has no means of distinguishing one type of non-central force from the other. However, that is contrary to observations. But how could the Moon possibly know when to cancel a non-central force component, and when to respond to it?

The only logical answer to this dilemma is that no such mysterious, deus ex machina force exists because gravity has no aberration in need of canceling. In that case, we may be certain that gravity propagates much faster than lightspeed.

9. Conclusions

The evidence from all six experiments that bear on the question of the speed of gravity is unambiguous in excluding answers as slow as lightspeed. A similar remark applies to the propagation speed of electrodynamic forces. The strongest of these experiments sets a lower limit to the speed of gravity of 2x1010 c. All objections and questions about this conclusion raised during the last two years have now been addressed and answered. In particular, claims (championed by Steve Carlip) that such a result is inconsistent with general relativity are now shown to be false. Moreover, no serious claim of experimental support for gravity propagating at lightspeed has been advanced in modern times.

When a source mass accelerates, that induces changes in its gravitational field. The lack of detectable aberration (propagation delay) for those changes means that the distant gravitational field accelerates when the source mass accelerates, in lockstep. To avoid direct violation of the causality principle, the propagation delay must be finite, even though much smaller than the corresponding propagation delay for photons. Because special relativity (SR) forbids propagation speeds faster than lightspeed in forward time, that theory is in conflict with, and is potentially falsified by, this result. GR has always implicitly recognized these facts through its equations of motion, which use instantaneous coordinates and momenta rather than retarded ones. That and its reliance on a single frame to define “coordinate time” mean that GR is based as much on Lorentzian relativity (LR) as on SR. These two theories, LR and SR, both employ the relativity principle and the same math (Lorentz transformations), but LR adopts a preferred frame and lacks the reciprocity between frames postulated by SR. Interestingly, no experiment testing SR or LR confirms frame reciprocity, yet all experiments are consistent with LR. [18] Therefore, the falsification of SR in favor of LR has no mathematical consequences for GR. The main physical consequence is negation of the proof that faster-than-light propagation is impossible.

Because of the belief that GR is based on SR, which disallows the possibility of faster-than-light propagation in forward time, the most common interpretation of GR is that the speed of gravity is the speed of light. This interpretation is also based on a misunderstanding of the implications of aberration and confusion between the meanings of gravitational force variations and gravitational waves. However, the consequences of a propagation speed of gravitational force variations as slow as lightspeed would be catastrophic for many astrophysical bodies, and are strongly disallowed by physical principles and by all existing experimental evidence.

The following is the single, most important conclusion to be noted here, and would be true even if the most common interpretation of GR could be shown to be consistent with all experimental evidence and with a gravity propagation speed of c. The mere existence of a viable alternative interpretation of the GR equations based on Lorentzian relativity, taken together with the continued experimental viability of LR, mean that the proof of the impossibility of propagation and communication in forward time at arbitrarily high speeds no longer has supportable experimental underpinnings.

Version of 2000/08/12

 

[1] T. Van Flandern, Phys. Lett. A 250 (1998) 1-11.
[2] D.M. Greenberger, A.W. Overhauser, Sci.Amer. 242 (May 1980) p. 66.
[3] C.W. Sherwin, R.D. Rawcliffe, Report I-92 of March 14, 1960 of the Consolidated Science Laboratory, Univ. of Illinois, Urbana; obtainable from U.S. Department of Commerce’s Clearinghouse for Scientific and Technical Information, document AD 625706.
[4] T.E. Phipps, Jr., Heretical Verities, Classic Non-fiction Library, Urbana (1986) 273-282.
[5] L.J. Wang, A. Kuzmich, A. Dogariu, Nature 406 (2000).277-279.
[6] W. Heitmann, G. Nimtz, Phys. Lett. A 196 (1994) p. 154.
[7] P. Weiss, Sci. News 157 (2000) p. 375.
[8] T. Van Flandern, Meta Res. Bull. 9 (2000) 1-9, see <http://metaresearch.org>.
[9] R.M. Wald, General Relativity, U. of Chicago Press, Chicago (1984) p. 67.
[10] R.P. Feynman, Feynman Lectures on Gravitation, Addison-Wesley, New York (1995) p. 113.
[11] C.W. Misner, K.S. Thorne, J.A. Wheeler, Gravitation, W.H. Freeman & Co., San Francisco (1973) p. 1095, p. 177.
[12] G.E. Marsch, C. Nissim-Sabat, Phys. Lett. A 262 (1999) 103-106.
[13] M. Ibison, H.E. Puthoff, S.R. Little, < http://xxx.lanl.gov/abs/physics/9910050>.
[14] T. Van Flandern, Phys. Lett. A 262 (1999) 261-263.
[15] S. Carlip, Phys. Lett. A 267 (2000) 81-87.
[16] A. Eddington, Space, Time & Gravitation, 1920; reprinted by Cambridge Univ. Press (1987) p. 109.
[17] F. de Felice, Gen. Rel. & Grav. 2 (1971) 347-357.
[18] T. Van Flandern, Open Questions in Relativistic Physics, F. Selleri, ed., Apeiron, Montreal (1998) 81-90.
[19] R. Mansouri, R.U. Sexl, Gen.Rel.&Grav. 8 (1977) p. 497.
[20] A. Einstein, L. Infeld, B. Hoffmann, Ann. Math. 39 (1938) 65-100.
[21] H.P. Robertson, T.W. Noonan, Relativity and Cosmology, W.B. Saunders Co., Philadelphia (1938).
[22] J.M.A. Danby, Fundamentals of Celestial Mechanics, Willmann-Bell, Richmond (1988) p. 125.
[23] D. McCarthy, This email address is being protected from spambots. You need JavaScript enabled to view it., USENET discussions in sci.physics.relativity (April 2000).